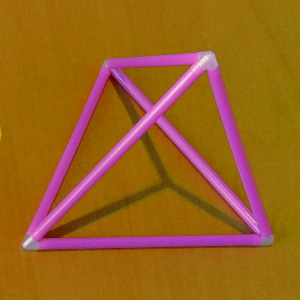




A tetrahedron is a specific Platonic solid. In addition to having all faces and edges the same, it also has the property that it is dual to itself, meaning the number of its vertices and faces is the same. This property can be used to derive its construction from a hexahedron (cube). By connecting the equally spaced vertices of a hexahedron, you get two tetrahedra that are mutually dual.
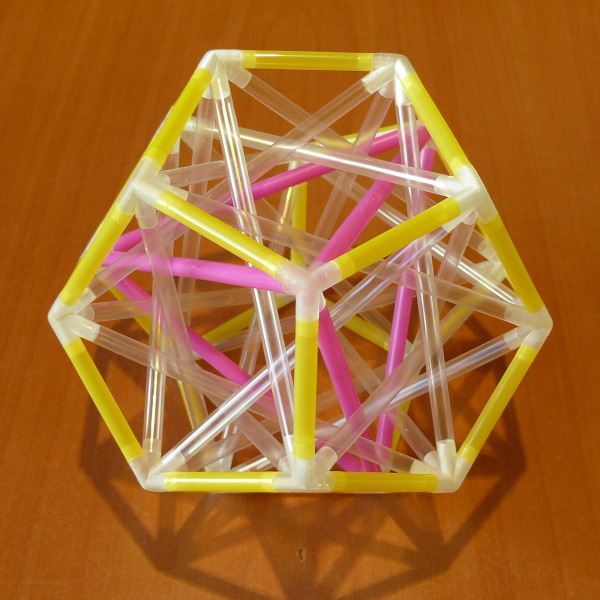


Another way to create a tetrahedral structure is from a dodecahedron. Again, if you connect equally spaced vertices of a dodecahedron, you get ten tetrahedra. These ten tetrahedra can be divided into two groups of five, where in each group, each vertex is part of exactly one tetrahedron. If you choose the right diameter for all edges (in relation to the edge length, it is 0.07383), you can assemble these five constructions in such a way that they touch each other, and this compound is stable because all tetrahedra fix each other. For a specific edge diameter, the operation will be reversed - you will divide the diameter by the number 0.07383 (multiply it by 13.5441 = 1/0.07383).
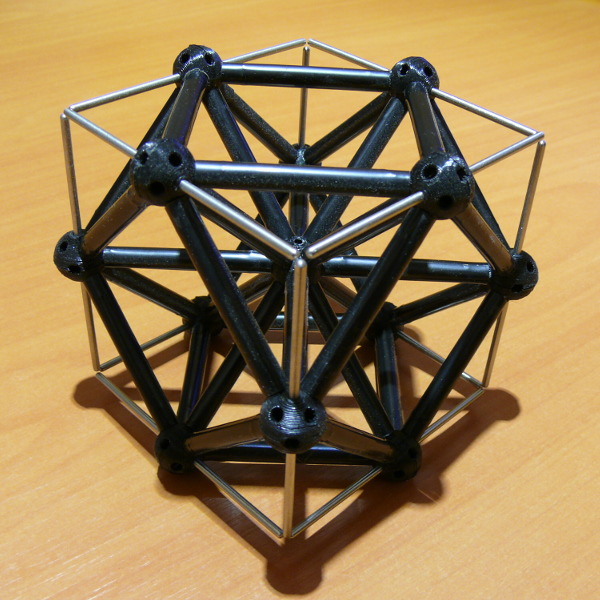

An interesting property of the tetrahedron is that it complements the octahedron excellently. These are the only two Platonic, meaning perfectly regular, solids that can be periodically connected to each other to completely fill 3D space. In other words, if we connect one octahedron with four tetrahedra, we get a structure, a building block, that can be periodically connected in space without any leftover space. The tetrahedron, as the strongest structural element, provides strength to this filling. Moreover, the construction of the tetrahedron can also be derived from the octahedron, precisely because it is its complement.



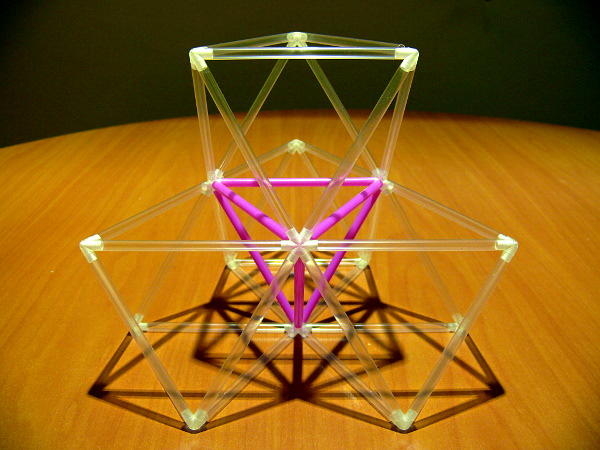
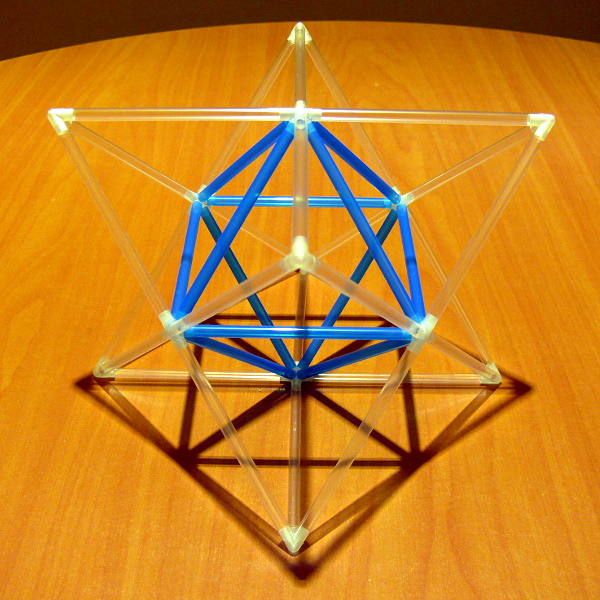
If we put together four equally oriented octahedra so that they enclose a space between them, this space will have the exact shape of a tetrahedron. The reverse is also true. Eight tetrahedra can be put together in such a way that the space between them forms an octahedron.

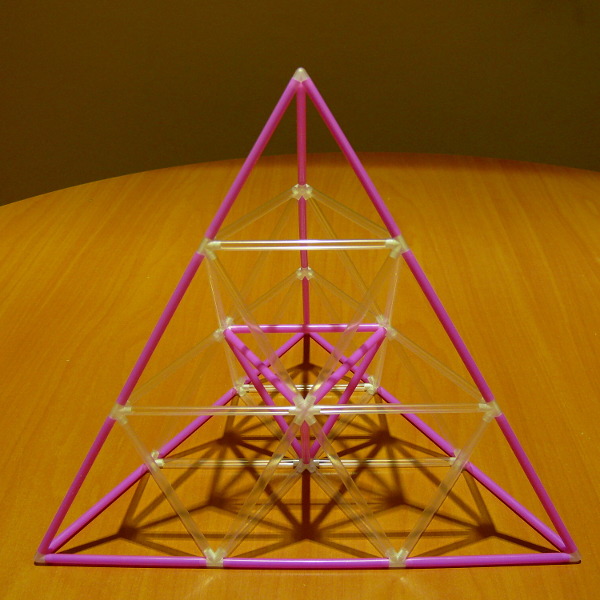
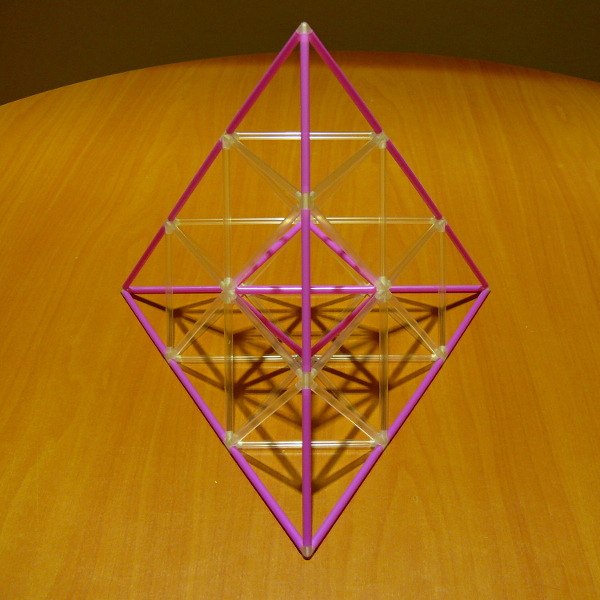
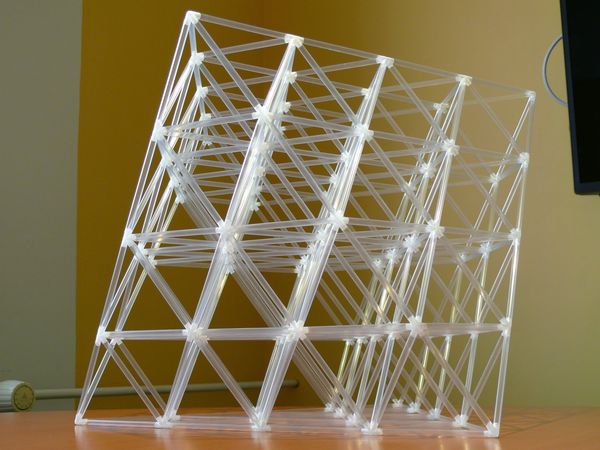
The duality of solids can be demonstrated by connecting their edges. In the case of lattice models, this is a relatively simple solution because all their edges just need to be divided in half by a new vertex, which will always be the center of a right-angle cross. Another option is to demonstrate duality by embedding one solid into another in such a way that the vertices of the embedded solid are placed precisely in the centers of the faces of the original solid. For lattice models, we then need to create a "scaffold" for these centers, which is slightly more complicated. For the tetrahedron, we can do this by dividing each face (facet) into 9 smaller ones, creating a central vertex. Edges will be divided into 3 smaller ones. If we connect all the neighboring vertices created in this way, the "scaffold" for the embedded tetrahedron will consist exactly of 4 octahedra and 10 tetrahedra of the same size as the embedded one.

