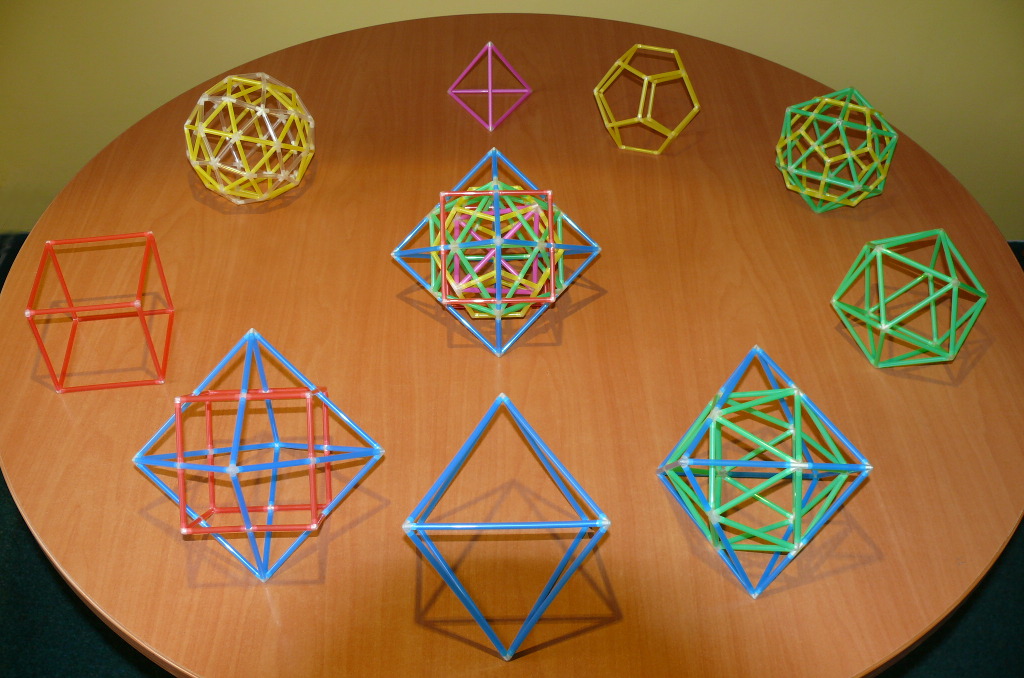
Mathematical principles are universal and valid for "ages of ages." One of the most famous thinkers who identified the perfection of some shapes in the real world was Plato, whose name these shapes began to be called by. Maybe he wasn't even the first to "discover" these shapes, but he certainly contributed to popularizing them with the stamp of his much more well-known philosophical view of the world. Many other thinkers followed, captivated by the perfect regularity and mathematics of these geometric figures, and attempted to expand this topic into greater breadth, deriving many other interesting shapes. However, in our three-dimensional world, there are only 5 perfectly regular ones.
Their regularity lies in the fact that all edges and faces of the shape are perfectly identical (lengths, angles, symmetry). From a more general perspective, this set can be limited to 3 basic shapes (octahedron, icosahedron, tetrahedron), as two of them (dodecahedron and hexahedron) can be easily derived by the principle of duality. All edges of the derived/dual shape are perpendicular tangents to the edges of the original shape. By using a different principle, namely the coincidence of octahedral planes with the "octant" planes of the icosahedron, we can easily derive one dual pair from the other, i.e., the pair octahedron-hexahedron to the pair icosahedron-dodecahedron. From one initial shape (octahedron), we can derive a total of four shapes (octahedron, hexahedron, dodecahedron, and icosahedron) with just two mathematical principles.
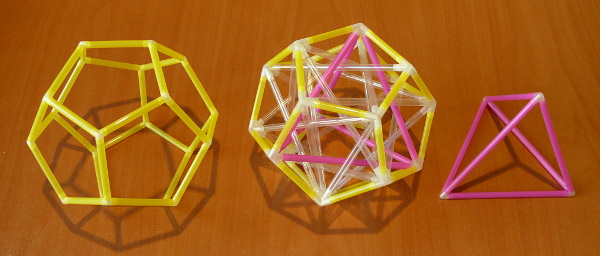
The tetrahedron, the fifth Platonic solid, can be derived from any pair by selectively connecting four equally distant vertices of the less-faced representative each time.
Using these principles, we can integrate all five Platonic solids into one construction. The first image shows five individual constructions, four transitional/derivative ones, and one integral one in which all solids are combined. There is also one additional construction, which, while not belonging to the Platonic solids, is derived from the icosahedron by "breaking edges." It is an equally significant geodesic dome, which is another ideal candidate for a solid of higher "roundness." The tetrahedron, octahedron, and icosahedron are, in the virtual "facet" world, "spheres," just with different degrees of "roundness." There is no other perfectly regular solid beyond these, but if we start from the "most spherical" icosahedron, by dividing/rounding its facets, we obtain a solid that is equally regular but "rounder" (by symmetry). That's why it is widely used wherever a sphere needs to be "described" by facets. For more information, see Geodesic Dome.
All the constructions mentioned above are excellent examples for demonstrating the use of the project (transforming an object - a network of nodes and edges - from a virtual form to a real one) and at the same time, a practical demonstration of the perfection of mathematics. It is not necessary to know all the mathematical abstractions and principles. Just take a look at the constructions and try to discover them yourself. Even mathematics can be fun.
Edges: any rod or tubular material (demonstrated here with plastic straws).
Nodes: 3D printed using any applicable and available technology (here, stereolithography was chosen).
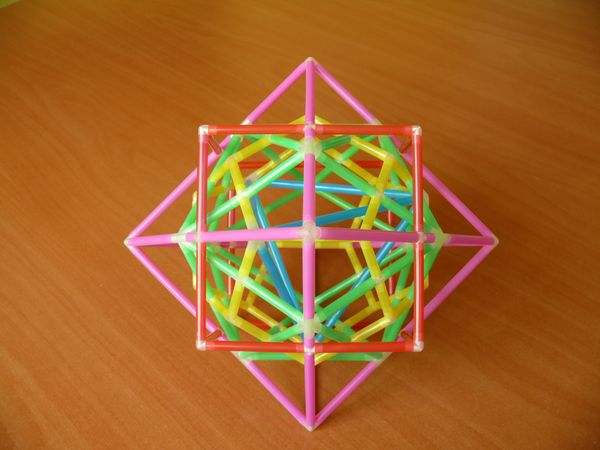
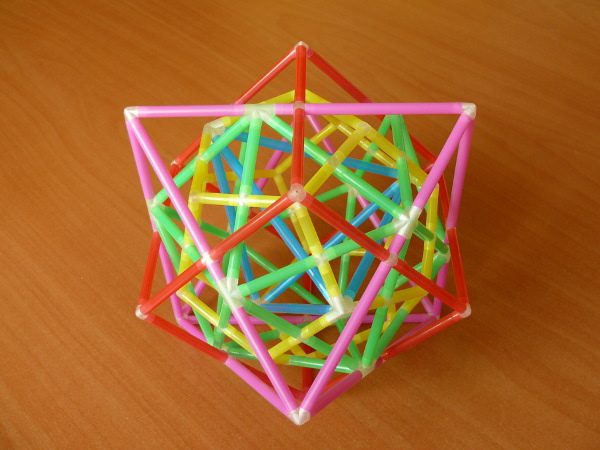
In the first version of the model construction (composition of all Platonic solids together), there was an error in designing the colors of the individual edges, and also in assembly, where there was not just one possible solution for the set of parts. In fact, there are exactly two. Unfortunately, the less symmetrical one was chosen during assembly. The tetrahedron and dodecahedron are incorrectly assembled with the icosahedron in the construction. This disrupts the maximum possible symmetry of the composition.
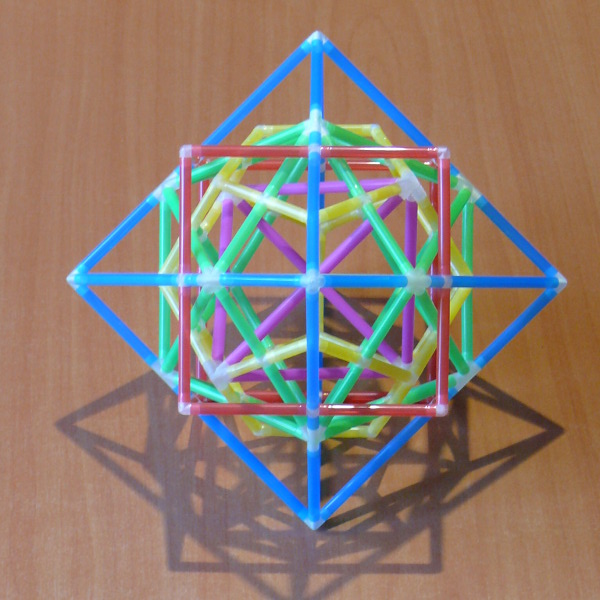
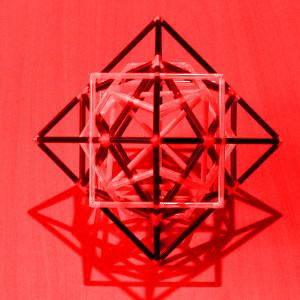
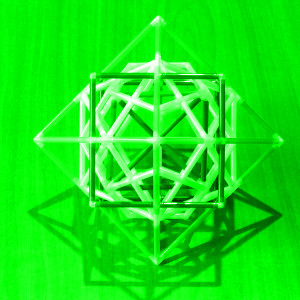

In the second version, the composition is assembled correctly, and the colors have been adjusted for better differentiation of individual solids. The colors of the tetrahedron and octahedron are swapped, which also better distinguishes the individual solids. This is most noticeable in the individual color channels.

