
The octahedron is one of the Platonic solids. It is structurally very simple. All you need to do is place two points on each of the three axes of the 3D coordinate system at the same distance from its center (0,0,0). One on the positive part of the axis and the other on the negative part. This will create 6 vertices of the octahedron, and by connecting them (except for the opposing ones), the edges of the octahedron will be formed. The edges will create equilateral triangles, which are also the eight faces of the octahedron. From it, by three simple operations, you can derive the other four Platonic solids. This is why the octahedron has a special position in experiments with the construction system.
Another, more complex, but possible way to derive all the Platonic solids is to start with the construction of any of them, and then derive the octahedron from it through operations, and from it, the rest.

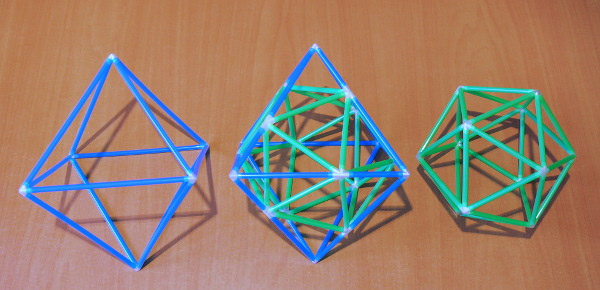
Polymer of 6 Octahedra
Some constructions can be periodically repeated and connected to each other. The octahedron is an ideal example of this, as it can be repeated in all directions without limitation. The period of repetition is not only directional, but also in size, where the polymer is a multiple enlargement of the original solid.
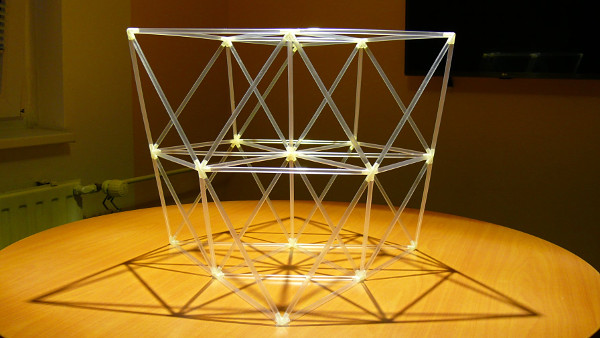



For finding the correct technique for connecting nodes and edges, it is necessary to choose a suitable construction. The technique is important from the perspective of the structural characteristics of the construction. For this purpose, the octahedron was chosen, which can be regularly "polymerized" - composed periodically in all directions while maintaining the parallelism of the surfaces. At the same time, it forms quadrilaterals in the remaining spaces of the construction (after subtracting the octahedra). The quadrilateral is the most stable regular polyhedron. Its integration into constructions adds stability to them. Therefore, the most suitable candidate for stress tests was chosen to be the construction of the octahedron/quadrilateral polymer. Here, the test is demonstrated with a beer glass, gradually filled with empty bottles. Due to the lack of locked joints, the construction collapses before the end of the test by disconnecting some of the weakest joints and subsequently breaking the edges under the greatest pressure. If the joints were locked to the desired tension, the construction would likely withstand a much greater load.





Polymer of 44 Octahedra
The possibility to repeat the structure of the octahedron in all directions to infinity, while at the same time having a simple production of edges of one type and an assembly technique that can be periodically automated, provides space to verify permissible tolerances in the length of edges, which is cumulative. The construction with a greater number of periods and greater deviations in the length of individual edges may be infeasible. For the polymer of 44 octahedra with edges of 5 mm in diameter, a tolerance of 0.3 mm is acceptable.
Edge:
- Material: Plastic straw
- Diameter: 5 mm
- Length: 91 mm
- Types: 1
- Quantity: 360
- Production: Hand cutting
Node:
- Material: Resin
- Locks: No
- Types: 4
- Quantity: 85
- Production: 3D printing
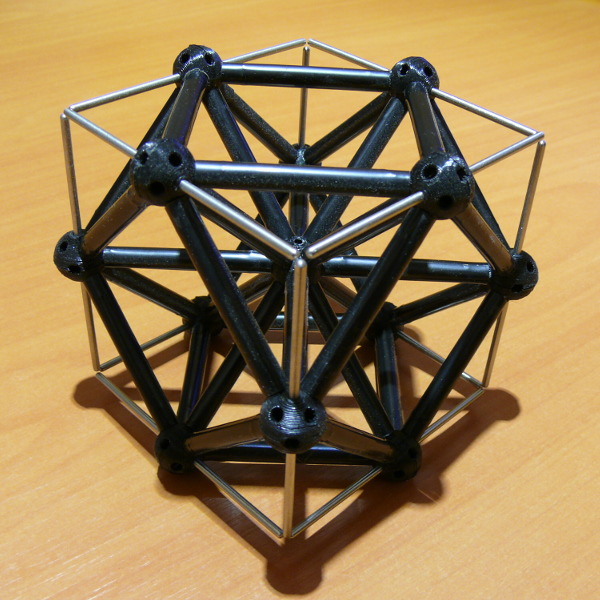
For the initial tests of the system, a construction based on an octahedron trimmed to a dual hexagon was used. The nodes are composed of two halves printed with PLA filament. The models of the nodes are enriched with a spherical body, which serves as support for internal connections of the edges of the hexagon/cube (metal rod 2.5 mm). Other connections are external (pins, hidden inside the edges in the image) and have a hole extending through the entire node to the opposite side. The hole is prepared for a screw lock (variant of aluminum edges), but in this PLA version and size, the proposed lock solution proved to be unsatisfactory. Finally, only edges made of a plastic straw with a diameter of 6 mm were used. Many thanks for the dedicated collaboration in "filament" printing go to Michal Jirásek, who very willingly helped in exploring the possibilities of 3D printing using FFF/FDM technology. Filament printing is suitable only where long printing times are not a problem.


For a random test of the lower limit of node size, empty pen ink supplies were used. Their outer diameter is approximately 3 mm and the inner diameter is 1.3 mm. Printing nodes from standard resin is problem-free, but a pin with a diameter of 1.3 mm is too fragile for assembling more complex constructions. This problem can be solved by choosing a different type of resin. There are also stronger and more flexible variants available.

